Question
Why is sampling a critical step in large-scale data
analysis?Solution
Explanation: Sampling is a critical step in data analysis, especially when dealing with large datasets. By selecting a smaller, representative subset of the population, analysts can perform computations faster and with fewer resources while retaining the integrity of the results. Effective sampling reduces data complexity and ensures that the sample mirrors the population's characteristics, enabling reliable statistical inferences. Techniques like simple random sampling or stratified sampling ensure diversity and minimize bias, maintaining the overall data quality for analysis. Option A: While sampling aids in efficient predictions, it does not involve using all available data. Sampling is about selecting a subset, not the entire dataset. Option C: Sampling does not eliminate the need for advanced techniques; it complements them by making analysis feasible for large datasets. Option D: Including all rare events is not guaranteed through sampling; specific strategies like oversampling are needed for rare-event analysis. Option E: Sampling reduces but does not eliminate bias. Proper technique selection minimizes it.
Solve for x in the equation: 3(x + 2) + 2(2x - 5) = 5x + 9
A solid metallic sphere of radius 15 cm is melted and recast into spherical balls of radius 3 cm each. What is the ratio of the surface area of the orig...
If
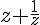 = 2 then find
= 2 then find The coefficient of ‘b2’ in (3a + b)3 is
If (x 2 = 10x – 25), then find the value of [x³ + (x⁴/25)][7 - x³]
(√ (sec2 θ + cosec2 θ )) ((sinθ (1 + cosθ ))/(1 + cos&thet...
Find the largest 5-digit number that is divisible by 12, 15, 18, and 24. Also, what is the remainder when this number is divided by 11?
(15 x 15 x 15 + 8 x 8 x 8)/(15 x 15 -15 x 8 + 8 x 8) = ?
If 8x3 - 125y3 = (2x - Ay) X (Bx2 + 25y2 + Cxy), then find the value of 3 X (2A + 6B) - 2C.


