Question
Find the equation of the circle passing through the
points (1, 2) and (2, -1), and whose center lies on the line x - 2y + 3 = 0.Solution
Let the center of the circle be (h, k), and the equation of the circle be (x - h)^2 + (y - k)^2 = r^2. Since the circle passes through (1, 2) and (2, -1): (1 - h)^2 + (2 - k)^2 = r^2 and (2 - h)^2 + (-1 - k)^2 = r^2. Also, the center lies on the line x - 2y + 3 = 0, so h - 2k + 3 = 0. Solve this system of three equations to find h, k, and r. After solving: h = 1, k = -2, and r^2 = 8. The equation of the circle is (x - 1)^2 + (y + 2)^2 = 8.
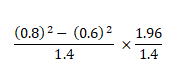
If a, b and c are integers such that a 2 + b 2 + c 2 = 228, a + b + c = 26 and b = c, then find the value of a?
If 2x+1, x+2, 2 and 5 are in proportion, then what is the mean proportional between 3.5(1-x) and 8(1+x)?
- If (x - y) = 4, then find the value of (x³ - y³ - 12xy).
If tan x + cot x = 7, find tan3 x + cot3 x = ?
If x = 35, then the value of x5 - 36x4 + 36x3 - 36x2 + 36x - 1 is
Given a triangle with two sides measuring 12 cm and 16 cm, which of the following cannot be a possible length for the third side?
A carriage travelling in fog passed along a man who was walking @ 6 km/hr in the same direction. He could see the carriage for 8 minutes and it was visi...
Atul has Rs.500 with him. He invested 30% of the amount at 5% p.a. for 6 years and rest at 20% p.a. for 4 years. Find the sum of simple interests receiv...


