Question
What is the area of the circular Track?
Statement-I: The area of equilateral triangle that can be inscribed in circular Track is 48√3 m2 Statement-II: The area of square of maximum size that can be inscribed in a circular Track is 196 m2. The question consists of two statements numbered “I and II” given below it. You have to decide whether the data provided in the statements are sufficient to answer the question. A. The data in statement I alone are sufficient to answer the question, while the data in statement II alone are not sufficient to answer the question. B The data in statement II alone are sufficient to answer the question, while the data in statement I alone are not sufficient to answer the question. C The data either in statement I alone or in statement II alone are sufficient to answer the question. D The data given in both statements I and II together are not sufficient to answer the question. E The data in both statements I and II together are necessary to answer theSolution
Statement I:Area of equilateral triangle = 48√3 m2. (√3/4) × (side) 2 = 48√3 Side = √192 = 8√3 m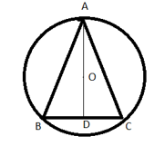 
Statement I: Area of equilateral triangle = 48√3 m2. (√3/4) × (side) 2 = 48√3 Side = √192 = 8√3 m Therefore, AD = √[(8√3)² – (4√3)²] = √144 = 12 m So, radius of circular field = OA = (2/3) × 12 = 8 m Therefore, area of the circular garden = πT × 8 × 8 = 64π m² Therefore, the question can be solved using statement-l alone. Statement-II: Are of square = 196 m² So, side of square =√196 = 14 m So, diagonal of square = √2 × side of square = 14√2 m Also, diagonal of square = diameter of circular garden So, radius of circular garden = 14√2/2 = 7√2 m Therefore, area of circular garden = π (7√2)² = 308 m² Therefore, questions can be solved using statement-ll alone. Hence, option c.
Where is the headquarters of the small finance bank which launched Platina Fixed Deposit, which offers interest of 15 basis points in addition to the r...
Recently Competition Commission of India (CCI) imposed a penalty of Rs 1,337.76 crore on which global tech firm for allegedly misusing its dominant posi...
- At what discounted price will the Zero Coupon Bonds of ₹1 lakh face value be issued by Power Finance Corporation?
According to the recent leadership appointment at Slice Small Finance Bank, who has been appointed as the Managing Director and Chief Executive Officer ...
Where was India’s first Open-Air Art Wall Museum inaugurated?
Consider the following statements with respect to the report on Trends and Progress of Banking in India 2022-23 provided by RBI related to the Gross...
Neeraj Chopra has qualified for Paris Olympics 2024 with a throw of how many metres?
RBI has permitted banks from how many countries to open Special Vostro Rupee Accounts (SVRAs)?
The forest department of which state recently decided to uproot the invasive alien species ‘senna spectabilis’ trees?
Who inaugurated the Mahila Aarogyam Kaksh wellness space at Shastri Bhawan?
Relevant for Exams:
