ЁЯУв Too many exams? DonтАЩt know which one suits you best? Book Your Free Expert ЁЯСЙ call Now!

    Question

    Consider two concentric circles having radii 17 cm and

    15 cm. What is the length (in cm) of the chord, of the bigger circle, which is a tangent to the smaller circle?
    A 12 Correct Answer Incorrect Answer
    B 16 Correct Answer Incorrect Answer
    C 10 Correct Answer Incorrect Answer
    D 8 Correct Answer Incorrect Answer

    Solution

    Given: ┬а Radius of circles r1= 15 cm ┬а r2= 17 cm Property of tangent - A tangent is perpendicular to the center of the circle. Property of chord - A perpendicular line drawn from the center of the circle to the chord of the circle always divide the chord in half. 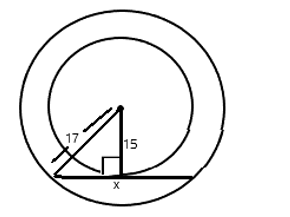Applying the property of chord and tangent: We find that- 172 = 152+(x/2)2 289 = 225 +x2 /4 289 тАУ 225 =x2 /4 x2 =256 x=16 ┬аTherefore, length of the chord = 16 cm.

    Practice Next
    More Geometry Questions

    Relevant for Exams:

    ask-question