Question
If in the given figure AD is perpendicular to BC, AC =
26 units, CD = 10 units, BC = 42 units, triangle DAC whose angle is x and angle B = y, then, find the value of 6/cosx -5/cosy +8 tany =?Solution
In ∆ ADC – AD = √(AC)2-DC2 ) AD = √(262-102 ) AD = √(676-100) AD = √576 = 24 AD = 24 Now in ∆ABD AB = √(AD)2+(BD)2 ) AB = √(242+322 AB = √(576+1024) = √1600 AB = 40 NOW- ∴The side opposite to the angle is the hypotenuse, so the base for angle x will be AD. cosx=24/26 cosy=32/40 tany=24/32 6/cosx -5/cosy +8 tany =? =6/(24/26)-5/(32/40)+8×24/32 =26/4 - 25/4 + 24/4 =(50-25)/4 =25/4 6/cosx -5/cosy +8 tany =25/4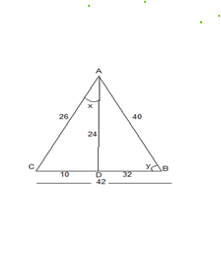
What was the theme of the 38th National Games of India?
How many additional capital buffers will HDFC Bank need to maintain as per the D-SIB framework from April 1, 2025?
What key role does KABIL aim to play through its MoU with CSIR-IMMT?
What is the disinvestment target set by the Government of India in Union Budget 2023-24?
Which of the following statements is/are NOT TRUE with respect to the UPI linkage by NPCI ?
I. The National Payments Corporation of India (NPCI...
CtrlS data centers has signed an MoU with which state government to set up an edge data center in the state with an investment of Rs 250 crore?
Which public sector bank recently inaugurated the ‘Ethical Hacking Lab’ at the Cyber Security Centre of Excellence (CCoE) in Hyderabad?
Match List-I (Scientist) with List-II (Field of Study) and select the correct answer using the codes given below:
Which organization did the Government of India sign a loan agreement with, amounting to 37 billion Japanese Yen (equivalent to $250 million), to fund th...
The Vietnam-India Bilateral Army Exercise (VINBAX) 2024 is being held in which Indian city?


