Question
The angle of elevation of the top of an unfinished tower at a point distant 78 m from its base is 30°. How much higher must the tower be raised (in
The angle of elevation of the top of an unfinished tower at a point distant 78 m from its base is 30°. How much higher must the tower be raised (in
m) so that the angle of elevation of the top of the finished tower at the same point will be 60°?
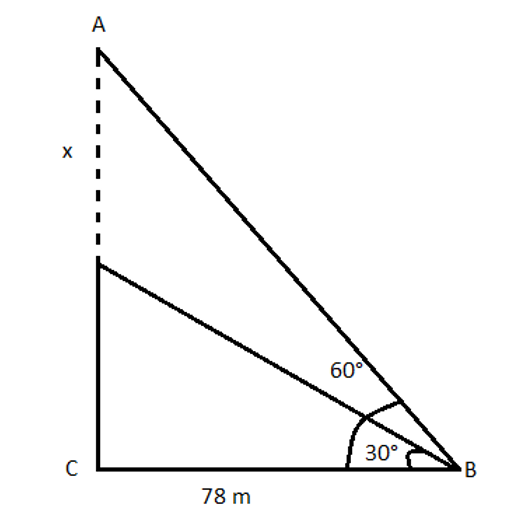Here, OC = Height of the unfinished tower AC = The height will be the finished tower's height In ΔOBC, tan 30° = OC/BC ⇒ 1/√3 = OC/78 [∵ tan 30° = 1/√3] ⇒ OC = 78/√3 ⇒ OC = (78 × √3)/(√3 × √3) = 26√3 .....(1) Let, the height of the tower will be raised (AO) = x In ΔABC, tan 60° = AC/BC ⇒ √3 = (OC + x)/78 [∵ tan 60° = √3] ⇒ x + OC = 78√3 ⇒ x + 26√3 = 78√3 ⇒ x = 78√3 - 26√3 = 52√3More Mensuration Questions
- A cylindrical water tank has a radius of 7 m and a height of 10 m. How much water will the tank hold in liters?
- O is the Centre of the circle passing through the points A, B and C such that ∠BAO=30 ° , ∠BCO=40 ° and ∠AOC=x ° , what is the value of x?
- A metal cylinder has a curved surface area of 528 cm² and its height is 12 cm. What is the diameter of the cylinder?
- If b sin θ = a, then sec θ + tan θ = ?
- Radius of a cylindrical vessel is 3cm less than breadth of rectangle, while height of cylindrical vessel is equal to length of rectangle, whose area is 720...
- The outer radius of a hollow metallic spherical ball is 12cm while the thickness of the metal used to make it is 8 cm. Find the volume of the metal used in...
- The lateral surface area of a cube is 484 cm2 . The length of a cuboid is 10% more than the side of the cube. The breadth of the cuboid is 9 cm less than i...
- The ratio of the radii of the bases of a cylinder and a cone is 4:5, while the ratio of their heights is 5:6. What is the ratio of the volume of the cylind...
- Two circles with centers B and D have radii DA = 8 cm and BC = x cm, respectively. AC is tangent to both circles. If DB and AC intersect the point E, AE = ...
- The length and breadth of a rectangle are increased by 8% and 5%, respectively. By how much percentage will the area of the rectangle increase?
Relevant for Exams:
Hey! Ask a query
Please enter email id
The email must be a valid email address.
Please enter Mobile Number
Please enter valid Mobile Number
Please enter your Doubt
