Question
The curved surface area of cylinder P is 16632
cm2 whose height and radius are (y+2) and (z-7) respectively. The radius of cylinder Q is 14 cm more than the radius of cylinder P. The curved surface area of cylinder P is 792 cm2 less than that of cylinder Q. If the height of cylinder Q is equal to the square of six, then which of the following statements is/are true? (i) The value of ‘y’ is the multiple of 7. (ii) The ratio between the volume of cylinder P and Q is (5y-11) : (3z+32) respectively. (iii) The value of [(difference between the values of ‘y’ and ‘z’)/10] is a prime number.Solution
The curved surface area of cylinder P is 16632 cm2 whose height and radius are (y+2) and (z-7) respectively. curved surface area of cylinder P = 2 x (22/7) x (z-7) x (y+2) = 16632 (44/7) x (z-7) x (y+2) = 16632 (1/7) x (z-7) x (y+2) = 378 (z-7) x (y+2) = 2646 Eq.(i) The radius of cylinder Q is 14 cm more than the radius of cylinder P. radius of cylinder Q = (z-7)+14 = (z+7) If the height of cylinder Q is equal to the square of six height of cylinder Q = 6x6 = 36 cm The curved surface area of cylinder P is 792 cm2 less than that of cylinder Q. The curved surface area of cylinder P is 16632 cm2 . 16632 = (curved surface area of cylinder Q) - 792 curved surface area of cylinder Q = 16632+792 = 17424 cm2 2 x (22/7) x (z+7) x 36 = 17424 (44/7) x (z+7) x 36 = 17424 (1/7) x (z+7) = 11 (z+7) = 77 z = 77-7 z = 70 Put the value of ‘z’ in Eq.(i). (70-7) x (y+2) = 2646 63 x (y+2) = 2646 (y+2) = 42 y = 42-2 y = 40 (i) The value of ‘y’ is the multiple of 7. The above given statement is not true. Because the value of ‘y’ is not the multiple of 7. (ii) The ratio between the volume of cylinder P and Q is (5y-11) : (3z+32) respectively. the ratio between the volume of cylinder P and Q = (5y-11) : (3z+32) = (5x40-11) : (3x70+32) = (200-11) : (210+32) = 189 : 242 So the volume of cylinder P and Q = [22/7 x (z-7) 2 x (y+2)] : [22/7 x (z+7) 2 x 36] Put the value of ‘y’ and ‘z’. = [22/7 x (z-7) 2 x (y+2)] : [22/7 x (z+7) 2 x 36] = [(70-7) 2 x (40+2)] : [(70+7) 2 x 36] = [(63) 2 x 42] : [(77) 2 x 36] = [63 x 63 x 42] : [77 x 77 x 36] = [9 x 9 x 7] : [11 x 11 x 6] = [9 x 3 x 7] : [11 x 11 x 2] = 189 : 242 The above given statement is true. (iii) The value of [(difference between the values of ‘y’ and ‘z’)/10] is a prime number. [(z-y)/10] = prime number [(70-40)/10] = prime number [30/10] = prime number prime number = 3 The above given statement is true.
Read the directions carefully and answer the following question.
If in the number ‘ 9887532691 ’, the even digits are shifted to the next d...
Select the options in which the numbers are related in the same way as are the numbers of the following set.
(541, 14, 737)
- This question is based on the five, three-digit numbers given below.
(Left) 847 538 628 482 497 (Right)
(Example- 847 – First digit = 8, ... How many pair of digits in forward and backward direction are there in the number “9257364” that has as many numbers between them as in the ...
If each of the letters in the English alphabet is assigned odd numerical value beginning A = 1, B = 3 and so on, what will be the total value of the let...
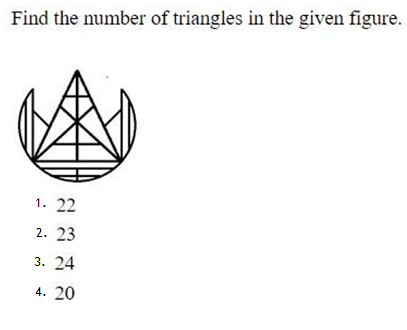
A series is given with one term missing. Choose the correct alternative from the given ones that will complete the series.
13, 38, 150, _____, 4478, 31341.
Select the number-pair in which the two numbers are related in the same way as are the two numbers of the following number-pair.
7 : 85
If 2 is subtracted from the second digits of each of the numbers, how many numbers thus formed will be divisible by three?
Two bus tickets from city A to B and three tickets from city A to C cost ₹ 77 but three tickets from city A to B and two tickets from city A to C cost...
Relevant for Exams:


