Question
What is the length (in cm) of chord PQ in a circle with
a radius of 7 cm, where a diameter AB and non- diameter chord PQ intersect perpendicularly at point C, and the ratio of AC to BC is 4:3?Solution
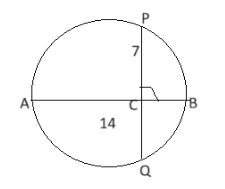Let AC = 4k and BC = 3k. Since C is the midpoint of AB (as AB is a diameter and C is where PQ perpendicularly intersects AB): AC + BC = AB Therefore, 4k + 3k = AB: 7k = AB Given AB = 2 × radius = 14 cm: 7k =14 k=2 Hence: AC =4k =4 × 2 = 8 cm,  BC=3k=3×2=6cm PC = CQ (AB is the diameter of given circle and PQ is the perpendicular on AB) As we know, PC × CQ = AC × CB PC × PC = 8 × 6  PC2 = 48  PC = √48  PC = 4√3 Now, PQ = 2×PC PQ = 2 × 4√3  PQ = 8√3
How many instrumental factors were used to compile Global Financial Centres Index (GFCI) 33?
A member of the IFSCA can resign from his office by giving in writing a notice to the Central Government of____________________
As per the SEBI (International Financial Services Centres) Guidelines, 2015 the stock exchanges operating in IFSC may permit dealing in ______________ t...
The Pension Fund Regulatory and Development Authority Act, 2013 came into force on ____________
Which of the following SEBI regulations are concerned with the issue of securities?
Philosophy that guides policy of an organization towards its employees and customers is a part of?
Which of the following is not one of the features of a Global Financial Centre?
Consider the following document and give the answer:
I.       Annual Financial Statement (AFS)
II.       Demands for ...
What is the role of political stability in the success of a global financial center?
A Banking Unit shall follow Know Your Customer norms, combating of financing of terrorism and other anti-money laundering requirements, including report...
Relevant for Exams:
