New 55% Off on All Courses - Enroll Now! Click Here

    Question

    There are a total of 42 students (boys and girls) in a

    class. The probability of picking at least one boy when two students are picked from the class is (195/287). Find the difference between the number of boys and girls in the class.
    A 8 Correct Answer Incorrect Answer
    B 9 Correct Answer Incorrect Answer
    C 5 Correct Answer Incorrect Answer
    D 6 Correct Answer Incorrect Answer
    E Cannot be determined Correct Answer Incorrect Answer

    Solution

    There are a total of 42 students (boys+girls) in a class. Let’s assume the number of boys in the class is ‘y’. number of girls in the class = (42-y) The probability of picking at least one boy from the class is 195/287. So  probability of picking at least one boy from the class = Probability of (1B, 1G or 2B)  out of 42 students = 195/287 { yc1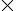  42-yc1  + yc2  } /  (42c2) = 195/287 [y(42-y)+{y(y-1)/2}]/861 = 195/287                   [Here 42C2 = 861] [y(42-y)+0.5y(y-1)]/3 = 195 y(42-y)+0.5y(y-1) = 585 42y-y2+0.5 y2-0.5y = 585 -0.5 y2+41.5y = 585 0.5 y2-41.5y = -585 0.5y2-41.5y+585 = 0 y2 - 83y+1170 = 0 y2 -(65+18)y+1170 = 0 y2 -65y-18y+1170 = 0 y(y-65)-18(y-65) = 0 (y-65) (y-18) = 0 y = 65, 18 As per the information given in the question, the total number of students is 42. So the value of ‘y’ cannot be 65. Because the number of boys cannot be more than the number of students in the class. So y = 18 number of boys in the class = 18 number of girls in the class = (42-y) = (42-18) = 24 So the difference between the number of boys and girls in the class = 24-18 = 6 Alternate Solution: If  the probability of picking at least one boy from the class is 195/287. then  The probability of picking no boy( both are  girls) from the class is  1- (195/287) = 92/287........(1) also let no of girls are "n"  so  The probability of picking no boy(both are girls) from the class is  nC2/42C2 = n(n-1)/{42 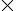41}  n(n-1)/(42 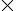41)= 92/287   (from  1) n(n-1) = 552 n = 24 no of boys = 42-24 = 18 So the difference between the number of boys and girls in the class = 24-18 = 6

    Practice Next

    Relevant for Exams: