Question
A train approaches a tunnel a PQ. Inside the tunnel a
cat located at a point i.e., 7/16 of a distance PQ measured from the entrance P. When the train whistles, the cat runs. If the cat moves to the entrance of the tunnel P, the train catches the cat exactly at the entrance. If the cat moves to the exit Q, the train catches the cat at exactly the exit. The speed of the train is greater than the speed of the cat by what order?Solution
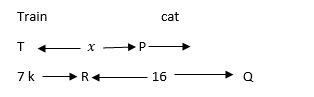Let the speed of train be ‘a’ and Speed of cat be ‘b’ and Train whistles at a point ‘T’, x km away from P, then First case: => a/b = x/(7k) Second case: => a/b = (x+16)/(9k) From both cases: => 9x = 7 (x+ 16 k) => 9x = 7x = 102 k => 2x = 102k => x/k = 56/1 => a/b = 56/(7x1) = 8/1
As per the Companies Act the Tribunal may at any time after passing of a winding up order, pass an order requiring any contributory for the time being...
The Advertisement of a Prospectus of a company shall specify therein the _____________
What is the word for "the study of ancient societies, cultures, and civilizations"?
The Seat of Supreme Court is discussed under which provision of the constitution of India?
For acquisition or holding of shares or voting rights in a target company, the acquirer have to make
Central Government has power to remove any difficulty arising in giving effect to the provisions of SEBI Act. What is the maximum period up to which su...
A minor may be admitted to the benefits of partnershipÂ
In a case involving the alleged murder of B by A, which of the following facts are relevant under Section 7 of Indian Evidence Act?
Which of the following entities is considered a "factor" according to the Factoring Regulation Act, 2011?
Why the Constitution is considered the supreme law of the land in India?
Relevant for Exams:
