Question
The angle of depression of vertex of a regular hexagon
lying in a horizontal plane, form the top of a tower of height 135 m located at the centre of the regular hexagon is 60°. What is the length of the each side of the hexagon?Solution
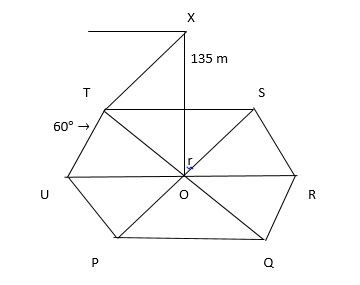Let OX be the height of the tower, angle of depression = Angle of elevation. y is the distance between u and o. In ∆XUO tan60° = 135/y √3 = 135/y y = 135/y ⇒ 45√3 In regular hexagon, ∆UOT, ∆ SOT,..... are equilateral triangles Length of hexagon = 45√3 m
- Determine the total sum of all three-digit numbers that are divisible by both 2 and 7.
Priya had 108 chocolates of two types – dairy and dark. If she receives 36 more dairy chocolates, the ratio of dairy to dark chocolates becomes 5:4. F...
Aman is forming pairs of two natural numbers, A and B, such that their product equals 350. He wants to determine how many such pairs exist where A and B...
Least number which when added to 9032 so that it is divisible by 76.
...Choose the number which is different from the group.
If (3 - 4/(1+ 2/(3+ 1/(2+1/3))))th part of a journey takes 45 minutes, then to complete 4/5th of that journey, it will take:
A six-digit number 4c68d2 is divisible by 12. Find the sum of all possible values of 'c' for the largest possible value of 'd'.
Aakriti’s present age is 5 times of Poonam’s age. The sum of the present ages of Aakriti, Poonam and Aakriti’s father is 86 yrs. Aakriti’s fathe...
How many terms are there in the geometric progression 7, 14, 28, ......, 14336?
In a class, 1/3 of the students are girls and rest are boys. If 3/5 of the girls and 1/5 of the boys are absent, what part of the total number of studen...
