Question
Four of the following five are alike in a certain way
as per the given arrangement and thus form a group. Find the one that does not belong to that group. Study the following information carefully and answer the question given below: Nine boxes viz. S, T, U, V, W, X, Y, Z and R are kept one above another but not in the same order. The box kept at the bottommost position is numbered as 1 and the box kept at the topmost position is numbered as 9. At most two boxes are kept below box U. Three boxes are kept between R and the box which is kept immediately above box U. W is kept two boxes above box R. Two boxes are kept between W and S. Box Z is kept immediately below box X. Box Y is kept three boxes below box X. Box T is kept above box V.Solution
We Have, At most two boxes are kept below box U. Three boxes are kept between R and the box which is kept immediately above box U.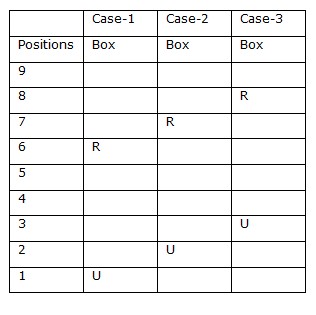 
Again we have, W is kept two boxes above box R. Two boxes are kept between W and S. 
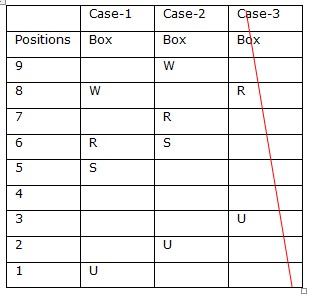Case-III gets eliminated because no space left for W. Again we have, Box Z is kept immediately below box X. Box Y is kept three boxes below box X. Box T is kept above box V. Case-I gets eliminated because no space left for X and Y. So final arrangement is Case-2 
In which Indian state is Kamlang Wildlife Sanctuary and Tiger Reserve located?
Which of the following things will RBI do if it has to combat raising inflation? Â
Central Depository Services Limited is promoted by which of the following:
Which state has set up India’s first bamboo village in it?
What instrument is Pandit Anindo Chatterjee renowned for playing?
For setting up TAFCUBs, in 2005 first MoU was signed with which state government?
__________ will invest up to Rs 60,000 crore to set up an advance semi-conductor manufacturing ecosystem in India over the next three years.
Which of the following scheme is launched to achieve electrification of every household by 2018
The 103rd Indian Science Congress was inaugurated on January 3, 2016 at university of _____
For an instant electronic fund transfer service that allows inter and intra-bank transfers within seconds to the customer account is a concept of?
Relevant for Exams:
