Question
How many seats are between M and K when counted from the
left of K? Study the following information carefully and answer the questions given below: A certain number of persons are sitting around the circular table. All of them are facing towards the center. Three persons sit between A and K who sits second to the left of C. E sits to the immediate left of G who sits second to the left of M. M sits third to the left of I who sits an immediate neighbour of K. Both E and A sit adjacent to each other. The number of persons sits between G and K is one less than the number of persons sits between K and E when counted from the right of both G and K respectively. At least one person sits between C and E when counted from the right of C.Solution
STEP I: M sits third to the left of I who sits an immediate neighbour of K. Three persons sit between A and K who sits second to the left of C. As per these statements, we can say that there are three possible cases and the arrangement will look like this: 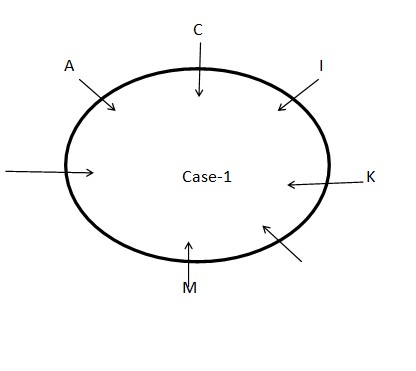
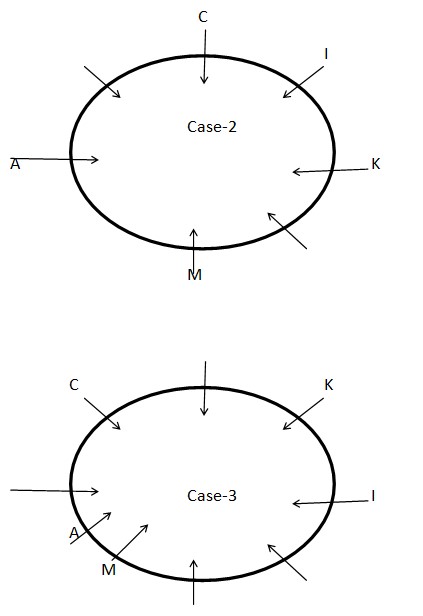 STEP II: E sits to the immediate left of G who sits second to the left of M. Both E and A sit adjacent to each other. The number of persons sits between G and K is one less than the number of persons sits between K and E when counted from the right of both G and K respectively. At least one person sits between C and E when counted from the right of C. As per these statements, CASE I and CASE III get eliminated and we continue with CASE II and the final arrangement will look like this:
STEP II: E sits to the immediate left of G who sits second to the left of M. Both E and A sit adjacent to each other. The number of persons sits between G and K is one less than the number of persons sits between K and E when counted from the right of both G and K respectively. At least one person sits between C and E when counted from the right of C. As per these statements, CASE I and CASE III get eliminated and we continue with CASE II and the final arrangement will look like this: 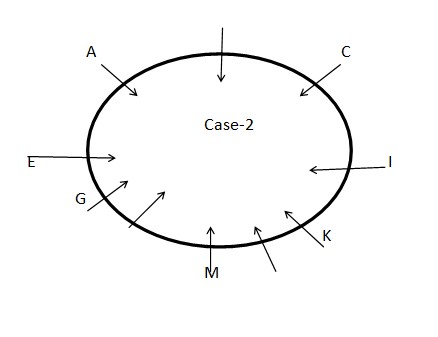
What does the term "derivative" include according to SCRA?
As per the provisions of the Companies Act every foreign company shall, within __________ of establishment of its place of business in India deliver to ...
As per provisions of CPC, the statement given by a judge is ______?
What is the sum which a company is required to deposit in the deposit repayment reserve account?
The plea can succeed only if it is shown that the accused was so far away at the relevant time that he could not be present at the place where the crime...
Who shall make rules as to cost of processes under Section 20 of the Court Fees Act?
Goods and Service Tax was added by which Amendment to the Constitution?
How many elected members are there in the Rajya Sabha?
Oral admissions as to the contents of a document are
The Pension Advisory Committee shall consist of______________
Relevant for Exams:


