📢 Too many exams? Don’t know which one suits you best? Book Your Free Expert 👉 call Now!

    Question

    Who sits 2nd to the left of

    U? Answer the questions based on the information given below. Ten people O, P, Q, R, S, T, U, V, W and X are seated in two parallel rows such that five persons are seated in each row but not necessarily in the same order. Persons seated in row 1 are facing towards the east direction while persons seated in the row 2 are facing towards the west direction. Therefore, in the given seating arrangement persons seating in row 1 are facing and sitting opposite to the persons seating in row 2 and vice versa. X sits at one of the extreme ends and sits three places away from the one who faces S. P faces the one who sits to the immediate left of S. Only one person sits between P and T, who faces Q. U sits second to the left of Q and is sitting adjacent to the one who faces R. T is not sitting adjacent to R. Neither O nor V sits at the extreme ends. V neither faces west nor sits adjacent to U.
    A Person who sits opposite to R Correct Answer Incorrect Answer
    B O Correct Answer Incorrect Answer
    C The one who faces P Correct Answer Incorrect Answer
    D X Correct Answer Incorrect Answer
    E None of the above Correct Answer Incorrect Answer

    Solution

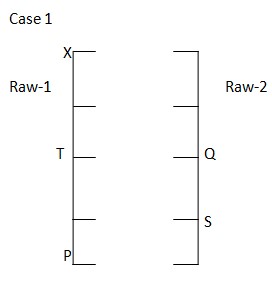
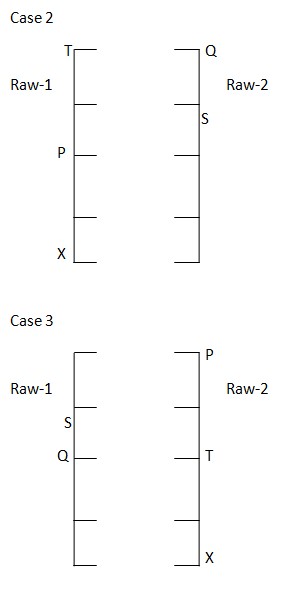
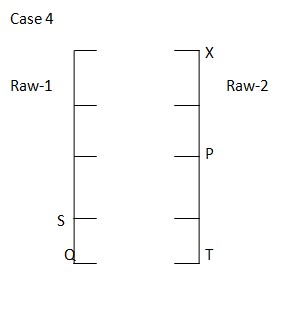
    X sits at one of the extreme ends and sits three places away from the one who faces S.P faces the one who sits to the immediate left of S. Only one person sits between P and T, who faces Q. Now, as X either sits extreme right or left end of row 1 or sits extreme right or left end of row 2 so, we have four possible places for X. U sits second to the left of Q and is sitting adjacent to the one who faces R.  T is not sitting adjacent to R.   case I and III are invalid as R and T are not sitting together. 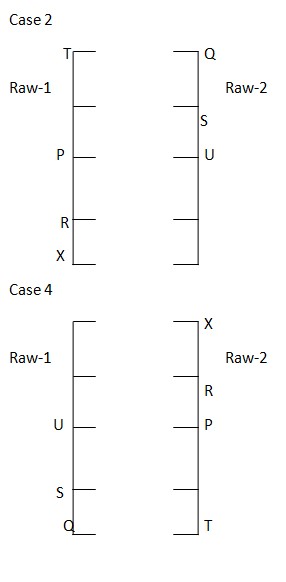Neither O nor V sits at the extreme ends.  V neither faces west nor sits adjacent to U.  case IV is rejected as we can’t fix V in case IV. The final arrangement is as follows:

    Practice Next
    More Seating Arrangement Questions

    Relevant for Exams:

    ask-question